Problem 1. Find
![]() for
for ![]() and
and ![]() . Then decide whether
the curve is concave up or down at
. Then decide whether
the curve is concave up or down at ![]() .
.
Answer. We have
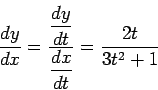
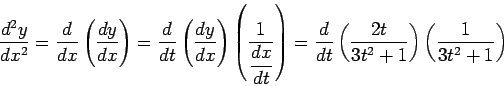
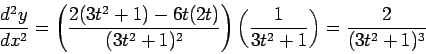
When ![]() , we get
, we get
Problem 2. Find the
indefinite integrals
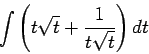
Answer. We have
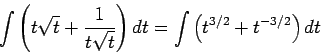

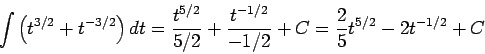
Problem 3. Find the definite integrals
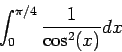
Answer. We have
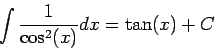
![\begin{displaymath}\int_0^{\pi/4} \frac{1}{\cos^2(x)}dx = \Big[\tan(x)\Big]^{\pi/4}_0 = \tan(\pi/4) - \tan(0) = 1-0 = 1\end{displaymath}](10-11-05/img18.gif)
Problem 4. Find the exact area below the
curve ![]() and above the x-axis.
and above the x-axis.
Answer. Graphing the function ![]() we see that
when
we see that
when
![]() the function is above the x-axis otherwise
it is below. And it crosses the x-axis when
the function is above the x-axis otherwise
it is below. And it crosses the x-axis when ![]() and
and ![]() . So
the area is given by the formula
. So
the area is given by the formula
![\begin{displaymath}\int_0^1 x^3(1-x) dx = \int_0^1 (x^3-x^4) dx = \left[\frac{x^4}{4} - \frac{x^5}{5}\right]_0^1 \end{displaymath}](10-11-05/img24.gif)
Problem 5. Find the derivatives
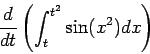
Answer. Recall the formula
